Physicists Designed a Quantum Rubik's Cube And Found The Best Way to Solve It

 Quantum physics already feels like a puzzle, but now scientists have made it more literal. A team of mathematicians from the University of Colorado Boulder has designed a quantum Rubik's cube, with infinite possible states and some weird new moves available to solve it.

The classic (and classical) Rubik's cube is what's known as a permutation puzzle, which requires players to perform certain actions to rearrange one of a number of possible permutations into a 'solved' state.

In the case of the infamous cube, that's around 43 quintillion possible combinations of small colored blocks being sorted into six, consistently-colored faces through a series of constrained movements.

But a quantum Rubik's cube cranks that possibility space up to infinity. All it takes is to give the solver a new quantum action – the ability to move a piece into a quantum superposition where it is both moved and not moved at the same time.

"With superpositions, the number of unique allowed states of the puzzle is infinite, unlike common permutation puzzles from toy stores," the researchers write in their paper.

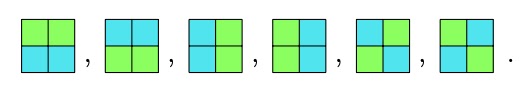
The team tested the idea on a simple version of a permutation puzzle: a two dimensional, 2×2 grid made up of just blue and green tiles. The solved state was to place the two green tiles above the two blue ones.

In its classical form, the puzzle only has six possible permutations, including the solved state. Any state can be transformed into any other through a sequence of swapping vertical and horizontal tiles – swapping diagonal tiles is forbidden, as is rotating the whole puzzle.

This basic puzzle can be given a quantum flavor by calling the colors 'particles,' and pointing out that because each tile is indistinguishable from the other of the same color, they are in a sense entangled.

Though the 'particles' have a quantum touch, in practice the puzzle itself is still played using classical moves. A truly quantum version opens up when superpositions between two different particles are allowed.

Three different types of simulated players were put to work solving the puzzle from 2,000 random scrambles. A classical solver's only move was to swap two adjacent tiles. A quantum solver could only enter pairs into quantum superpositions. And a combined solver could perform either action each time.

Unsurprisingly, the combined solver performed the best, solving the puzzle in an average of 4.77 moves. The quantum solver was next, with an average of 5.32 moves, while the classical solver came in last place with 5.88 moves on average.

That's not to say the realm of classical physics doesn't have its advantages though. The classical solver can actually reach the solution in fewer than five moves more often than the quantum solver. But it blows out its average because it can often take twice that long, where the quantum solver almost always finishes in eight moves or less.

This so-called quantum advantage should become more pronounced with more complex puzzles, the team says.

Noah Lordi, Akira Kyle, Josh Combes.

After a solver works through the permutations using their allowed moves – either classical, quantum, or both – the solution is then verified through a 'referee.'

If you're familiar with the old Schrödinger's cat thought experiment, you'll remember that the measurement itself causes the superposition to randomly become just one of the states. Ideally, that would be the solved state, but if not, the puzzle is scrambled again and the solver has to start over.

That's how the classical solver can even begin to tackle a quantum puzzle. Unless they get extremely lucky and the scrambled state is one of the six classical possibilities (out of infinite quantum options), they'll have to make moves that get them as close as possible to the solution, and hope that the measurement collapses the superposition into the solved state.

The six classical states of the 2×2 puzzle, with the solved state on the far left. (Lordi et al., Physical Review A, 2025)

Although the quantum solver seems to have the home ground advantage, it has one downside – it takes two moves for it to do a classical swap operation. That's how the classical solver gets an early head start in some versions of the puzzle, but why the combined solver always has the lead.

The team also went on to create a 3D version of the quantum puzzle, albeit not a full cube. It was 2x2x1 tiles, which also had infinite possibilities and could be solved through similar actions.

In practice, quantum permutation puzzles could potentially be built using arrays of ultracold atoms suspended in optical lattices. But mostly, it's a thought experiment for math nerds.

The research has been accepted for publication in the journal Physical Review A, and is currently available on the preprint server arXiv.